Select Chapter Topics:
Q. No.A point charge \(q\) is placed within a spherical cavity inside a metallic conductor, slightly off-centre. The charge \(q\) induces a charge distribution on the inner surface of the cavity. The net force on \(q\) due to the induced charges is:
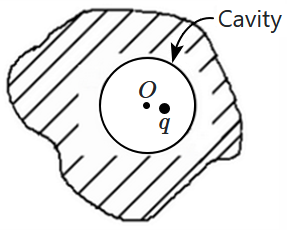
| 1. | zero |
| 2. | towards the centre |
| 3. | away from the centre |
| 4. | either towards or away from the centre depending on the sign of \(q\) |
Subtopic: Gauss's Law |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Three identical charges (\(q\) each) are placed at the three vertices \(A,B,C\) of an equilateral triangle of side \(a.\) Let \(O\) be the centre (centroid) of the triangle \(ABC\) and \(O'\) be the reflection of \(O\) in \(BC.\) The electric field at \(O,\) due to any one of the charges, is \(E.\) The net field at \(O',\) due to all the three charges, is:
| 1. | \(2E\) | 2. | \(\Large\frac{3E}{2}\) |
| 3. | \(\Large\frac{4E}{3}\) | 4. | \(\Large\frac{5E}{4}\) |
Subtopic: Electric Field |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Given below are two statements:
| Assertion (A): | The electrostatic field of a charge distributed uniformly over the surface of a sphere vanishes within the sphere, only at its centre. |
| Reason (R): | This cancellation occurs at the centre due to the symmetry of the sphere and the symmetric, uniform charge distribution. |
| 1. | Both (A) and (R) are True and (R) is the correct explanation of (A). |
| 2. | Both (A) and (R) are True but (R) is not the correct explanation of (A). |
| 3. | (A) is True but (R) is False. |
| 4. | (A) is False but (R) is True. |
Subtopic: Electric Field |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Which of the following field configurations is/are possible?
Note: \(A,B,C\) are conductors. Other charges may be present in the vicinity.
Note: \(A,B,C\) are conductors. Other charges may be present in the vicinity.
| 1. | I, III | 2. | II |
| 3. | I, II, III | 4. | none of I, II, III |
Subtopic: Electric Field |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Equal and opposite charges are placed at the two vertices of an equilateral triangle, giving a dipole moment \(p.\) A small dipole \(p'\) is placed at the third vertex, parallel to the previous dipole. If the electric fields due to both dipoles \(p,~p',\) at the mid-point of the dipole \(p,\) are equal, then \(\dfrac{p'}{p}\) equals:
| 1. | \(3\sqrt3\) | 2. | \(\dfrac{3\sqrt3}{2}\) |
| 3. | \(\sqrt3\) | 4. | \(\dfrac{\sqrt3}{2}\) |
Subtopic: Electric Dipole |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Match the following charge distributions with the corresponding expressions of their electric fields: \(\left(k={\dfrac{1}{4\pi\varepsilon_0}}\right)\)
| \(\mathrm{(A)}\) | A positive point charge \(q\) is placed at the centre of an uncharged conducting sphere. Electric field is measured outside the sphere, as a function of the distance \((r)\) from the centre. |
\(\mathrm{(I)}\) | \(E=0\) |
| \(\mathrm{(B)}\) | A positive point charge \(q\) is placed within an uncharged conducting sphere, but not at its centre. Electric field is measured outside the sphere, as a function of distance \((r)\) from centre \((O).\) |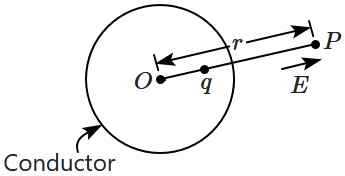
\(\mathrm{(II)}\) | \(E={\Large\frac{kq}{r^2}}\) |
| \(\mathrm{(C)}\) | A pair of point charges \((+q,-q)\) are placed within an uncharged conducting sphere, symmetrically about its centre \((O).\) 
Electric field is measured at \(P\) outside the conductor, but on the axis of the dipole. The distance \(OP=r.\) |
\(\mathrm{(III)}\) | \(E<{\Large\frac{kq}{r^2}}\) |
| \(\mathrm{(D)}\) | A positive point charge \(q\) is placed outside a spherical conductor, with the centre \(O.\)
Electric field \(E\) is measured at a point \(P,\) radially along the direction of the point charge from \(O,\) and the distance \(r\) is measured from \(q.\) |
\(\mathrm{(IV)}\) | \(E>{\Large\frac{kq}{r^2}}\) |
| 1. | \(\mathrm{A \text- I, B\text- I, C\text- III, D\text- IV}\) | 2. | \(\mathrm{A\text- II, B \text- IV, C \text- III, D \text- II}\) |
| 3. | \(\mathrm{A \text- II, B \text- II, C\text - I, D \text- III}\) | 4. | \(\mathrm{A \text- I, B \text- IV, C \text- I, D \text- IV}\) |
Subtopic: Electric Field |
58%
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
A thin uniform rod of mass \(M\) and length \(L\) is suspended from one of its ends, '\(A\)', so that it can rotate freely about it. A charge '\(q\)' is fixed to its lower end \(B\). A uniform horizontal Electric field is switched on and the rod rotates about \(A\), finally coming to equilibrium – making an angle of \(45^{\circ}\) with the vertical. If the acceleration due to gravity is '\(g\)', then,
1. \(qE =Mg\)
2. \(2qE =Mg\)
3. \(qE =2Mg\)
4. \(\sqrt{2}qE =Mg\)
1. \(qE =Mg\)
2. \(2qE =Mg\)
3. \(qE =2Mg\)
4. \(\sqrt{2}qE =Mg\)
Subtopic: Electric Field |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Two identical balls of cork \(\mathrm{(C_1,C_2)}\) are charged oppositely with equal amounts of charge distributed uniformly over their respective surfaces. Two similar (of same size) balls of copper\((M_1,M_2)\) are charged in the same way as the previous two balls. \(\mathrm{C_1,C_2}\) are allowed to touch each other briefly and are separated, and the same is done with \(M_1,M_2.\) The electrostatic force between \(\mathrm{C_1,C_2}\) is \(F_C\) and that between \(M_1,M_2\) is \(F_M\) at the same separation. Then:
| 1. | \(F_C=0,F_M\neq0\) | 2. | \(F_C\neq0,F_M=0\) |
| 3. | \(F_C=0,F_M=0\) | 4. | \(F_C\neq0,F_M\neq0\) |
Subtopic: Electric Charge |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
A dipole is placed at the centre of a uniformly charged circular ring, with the axis of the dipole along the axis of the ring.
The dipole experiences:
1. no force and no torque.
2. a force but no torque.
3. a torque but no force.
4. a force and a torque.
The dipole experiences:
1. no force and no torque.
2. a force but no torque.
3. a torque but no force.
4. a force and a torque.
Subtopic: Electric Dipole |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Given below are two statements:
| Statement I: | Gauss's law for electric fields is a consequence of the conservation of energy. |
| Statement II: | Coulomb's law for electric charges leads to a conservative electric field. |
| 1. | Statement I is incorrect and Statement II is correct. |
| 2. | Both Statement I and Statement II are correct. |
| 3. | Both Statement I and Statement II are incorrect. |
| 4. | Statement I is correct and Statement II is incorrect. |
Subtopic: Gauss's Law |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Select Chapter Topics:
© 2025 GoodEd Technologies Pvt. Ltd.