Select Question Set:
Q. No.The figure shows a current carrying loop having four segments \(1,\) \(2,\) \(3\) and \(4.\) The magnitude of the magnetic field at centre \(\mathrm{O}\) is maximum due to:
1.
segment \(1\)
2.
segment \(2\)
3.
segment \(3\)
4.
segment \(4\)
Subtopic: Biot-Savart Law |
84%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A straight current-carrying wire carrying current \(I\) passes perpendicular to the plane of an imaginary rectangular loop \(PQRS\), passing through its centre \(O\) (into the diagram). The diagonals intersect at \(60^\circ,\) and side \(PS\) is smaller than side \(PQ\). The value of \(\int \vec{B} \cdot d\vec{l}\) evaluated from \(P\) to \(Q\) (along \(PQ\)) has the magnitude:
| 1. | \(\dfrac{\mu_{0} I}{6}\) | 2. | \(\dfrac{2 \mu_{0} I}{6}\) |
| 3. | \(\dfrac{4\mu_{0} I}{6}\) | 4. | \(\dfrac{5\mu_{0} I}{6}\) |
Subtopic: Ampere Circuital Law |
52%
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Two long conductors, separated by a distance \(d,\) carry currents \(I_1\) and \(I_2\) in the same direction, exerting a force \(F\) on each other. Now, the current in one of the conductors is doubled and its direction is reversed, while the separation distance between the conductors is increased to \(3d.\) What is the new force between the conductors?
1. \(-2F\)
2. \(F/3\)
3. \(-2F/3\)
4. \(-F/3\)
1. \(-2F\)
2. \(F/3\)
3. \(-2F/3\)
4. \(-F/3\)
Subtopic: Force between Current Carrying Wires |
86%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
The strength of the magnetic field around a straight current-carrying conductor as observed by Biot and Savart was that:
| 1. | field is the same every where around the conductor. |
| 2. | field is directly proportional to the square of the current flowing in the conductor. |
| 3. | field obeys the inverse square law of distance. |
| 4. | magnetic field strength was maximum on the axis of the current conductor. |
Subtopic: Biot-Savart Law |
78%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A proton is rotating along a circular path with kinetic energy \(K\) in a uniform magnetic field \(B.\) If the magnetic field is made four times keeping the radius constant, then what will be the kinetic energy of rotation of the proton?
| 1. | \(16K\) | 2. | \(8K\) |
| 3. | \(4K\) | 4. | \(K\) |
Subtopic: Lorentz Force |
74%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A current-carrying wireframe is in the shape of digit eight \((8).\) It is carrying current \(i_0.\) If the radius of each loop is \(R_0,\) then the net magnetic dipole moment of the figure is:
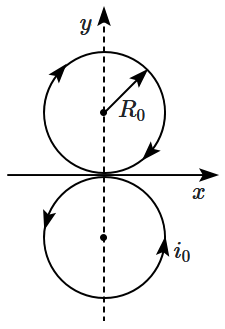
| 1. | \(\left({{i}_{0}\mathit{\pi}{R}_{0}^{2}}\right)\sqrt{2} \) | 2. | zero |
| 3. | \({i}_{0}\times{2}\mathit{\pi}{R}_{0}^{2} \) | 4. | \({i}_{0}\left({{4}\mathit{\pi}{R}_{0}}\right) \) |
Subtopic: Magnetic Moment |
89%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A current \(i\) flows through a semi-circular loop of radius \(r,\) attached to two long straight wires along the open diameter of the loop. The magnetic field at the centre of the loop is:
| 1. | \(\dfrac{\mu_0i}{4r}\) |
| 2. | \(\dfrac{\mu_0i}{4r}+\dfrac{\mu_0i}{2\pi r}\) |
| 3. | \(\dfrac{\mu_0i}{4r}+\dfrac{\mu_0i}{4\pi r}\) |
| 4. | \(\left[\left(\dfrac{\mu_0i}{4r}\right)^2+\left(\dfrac{\mu_0i}{4\pi r}\right)^2\right]^{\frac12} \) |
Subtopic: Magnetic Field due to various cases |
84%
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
A long solenoid of radius \(1~\text{mm}\) has \(100\) turns per mm. If \(1~\text{A}\) current flows in the solenoid, the magnetic field strength at the centre of the solenoid is:
| 1. | \(6.28 \times 10^{-4} ~\text{T} \) | 2. | \(6.28 \times 10^{-2}~\text{T}\) |
| 3. | \(12.56 \times 10^{-2}~\text{T}\) | 4. | \(12.56 \times 10^{-4} ~\text{T}\) |
Subtopic: Magnetic Field due to various cases |
66%
From NCERT
NEET - 2022
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
From Ampere's circuital law, for a long straight wire of circular cross-section carrying a steady-current, the variation of the magnetic field inside and outside the region of the wire is:
| 1. | A linearly decreasing function of distance upto the boundary of the wire and then a linearly increasing one for the outside region. |
| 2. | Uniform and remains constant for both regions. |
| 3. | A linearly increasing function of distance upto the boundary of the wire and then a linearly decreasing one for the outside region. |
| 4. | A linearly increasing function of distance \(r\) upto the boundary of the wire and then decreasing one with \(1/r\) dependence for the outside region. |
Subtopic: Ampere Circuital Law |
66%
From NCERT
NEET - 2022
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
The ratio of the radii of two circular coils is \(1:2.\) The ratio of currents in the respective coils such that the same magnetic moment is produced at the centre of each coil is:
| 1. | \(4:1\) | 2. | \(2:1\) |
| 3. | \(1:2\) | 4. | \(1:4\) |
Subtopic: Magnetic Moment |
63%
From NCERT
NEET - 2022
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Select Question Set:
© 2025 GoodEd Technologies Pvt. Ltd.