If two soaps bubbles of equal radii r coalesce  then the radius of curvature of interface between two bubble will be

1. r

2. 0

3. infinity

4. $\frac{r}{2}$

A cylinrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The  liquid emerging from the hole strike the ground at distance x

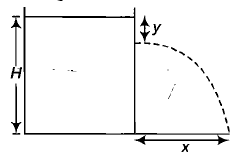
1. x is equal if hole is at depth y or H-y

2. x is maximum for y=$\frac{\mathrm{H}}{2}$

3. Both (1.) and (2) are correct

4. Both (1.) and (2.) are wrong

Water from a tap emerges vertically down with an initial speed of 10${\mathrm{ms}}^{-1}$. The cross-sectional area of tap is ${10}^{-4}{\mathrm{m}}^2$. Assume that the pressure is constant throughout the stream of water, and that the flow is steady. The cross sectional area of the stream 0.15 m below the tap is

1. $5.0\times {10}^{-4}{\mathrm{m}}^2$

2. $10\times {10}^{-5} {\mathrm{m}}^2$

3. $5.0\times {10}^{-5} {\mathrm{m}}^2$

4. $2.0\times {10}^{-5} {\mathrm{m}}^2$

A solid uniform ball of volume V floats on the interface of two immiscible liquids (Fig.) The specific gravity of the upper liquid is ${\mathrm{\rho }}_1$ and that of lower one is ${\mathrm{\rho }}_2$ whilst the specific gravity of ball is $\mathrm{\rho } ({\mathrm{\rho }}_1<\mathrm{\rho }<{\mathrm{\rho }}_2)$. The fraction of the volume of the ball in the upper liquid is

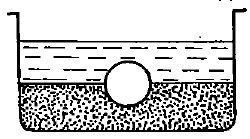
1. $\frac{{\mathrm{\rho }}_2}{{\mathrm{\rho }}_1}$

2. $\frac{{\mathrm{\rho }}_2-\mathrm{\rho }}{{\mathrm{\rho }}_2-{\mathrm{\rho }}_1}$

3. $\frac{\mathrm{\rho }-{\mathrm{\rho }}_1}{{\mathrm{\rho }}_2-{\mathrm{\rho }}_1}$

4. $\frac{{\mathrm{\rho }}_1}{{\mathrm{\rho }}_2}$

 A metal sphere connected by a string is dipped in a liquid of density $\rho$ as shown in figure. The pressure at the bottom of the vessel will be, ($p_0$atmospheric pressure)

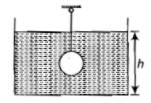
1. p=$p_0+\rho gh$

2. $p>p_0+\rho gh$

3. $p<p_0+\rho gh$

4. $p_0$

Two soap bubbles of radii ${\mathrm{r}}_1 \mathrm{and} {\mathrm{r}}_2$ equal to 4 am and 5 sm are touching each other over a common surface ${\mathrm{S}}_1{\mathrm{S}}_2$ (shown in figure). Its radius will be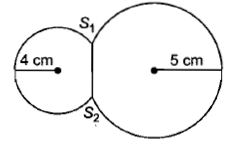

1. 4 cm

2. 20 cm

3. 5 cm

4. 4.5 cm

A piece of ice is floating in a beaker containing water when ice melts the temperature falls from 20$°$C to 4$°$C, level of water

1. remains uncharged

2. falls

3. rises

4. changes erratically

The work done in blowing a bubble of radius is W, then the work done in making a bubble of radius 2R from that solution is

1. $\frac{W}{2}$

2. 2W

3. 4W

4. $2^{\frac{1}{3}}W$

When the system shown in the adjoining figure is in equilibrium and if the areas of cross section of the smaller piston and bigger piston are a and 10 a mass on smaller and bigger pistons are  m amd M, then

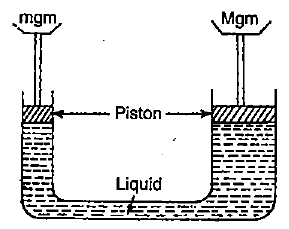
1. m=M

2. m= 10M

3. M= 10 m

4. M= 100 m

A wooden block, with a coin placed on its top, floats in water as shown in fig. the distance l and h are shown there. After some time the coin falls into the water. Then

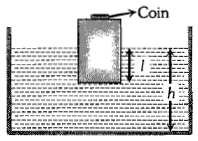
1. l decreases and h increases

2. l increases and h decreases

3. Both l and h increase

4. Both l and h decrease