Given,

q₁ = 5 x 10^-8 C

q₂ = -3 x 10^-8 C

Distance the two charges, d = 16 cm = 0.16 m

Consider a point P on the line joining the two charges, as shown in the given figure.

r = Distance of point p from charge q₁

Let the electric potential (V) at point P be zero.

The potential at point P is the sum of potentials caused by charges q₁, and q₂ respectively.

Therefore,  $V=\frac{1}{4\pi {ϵ}_o}.\frac{q_1}{r}+\frac{1}{4\pi {ϵ}_o}.\frac{q_2}{d–r}\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\mathit{ }\dots \dots \dots .\left(1\right)$

Where,

${\mathrm{\epsilon }}_0$​ = permittivity of free space.

Putting  V = 0, in eqn. (1), we get,

$0=\frac{1}{4\pi {ϵ}_o}\cdot \frac{q_1}{r}+\frac{1}{4\pi {ϵ}_o}\cdot \frac{q_2}{d-r}$
$\frac{1}{4\pi {ϵ}_o}\cdot \frac{q_1}{r}=-\frac{1}{4\pi {ϵ}_o}\cdot \frac{q_2}{d-r}\frac{q_1}{r}=-\frac{q_2}{d-r}\frac{5\times {10}^{-8}}{r}=-\frac{(-3\times {10}^{-8})}{0.16-r}$
$5(0.16-r)=3r$
$0.8=8r$
$r=0.1m=10cm$

Therefore, the potential is zero at a distance of 10 cm from the positive charge between the charges.

Suppose point P is outside the system of two charges at a distance s from the negative charge, where potential is zero, as shown in the following figure.

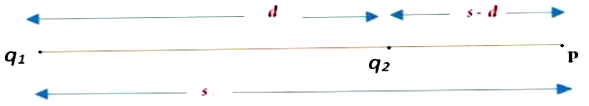
For this arrangement, the potential is given by,

$\mathrm{V}=\frac{1}{4{\mathrm{\pi ϵ}}_{\mathrm{o}}}.\frac{{\mathrm{q}}_1}{\mathrm{s}}+\frac{1}{4{\mathrm{\pi ϵ}}_{\mathrm{o}}}.\frac{{\mathrm{q}}_2}{\mathrm{s}–\mathrm{d}} \dots \dots \dots \dots \dots \dots \dots 2$

Where,

${\mathrm{\epsilon }}_0$​ = permittivity of free space.

For V = 0, eqn. (2) can be written as :

$\begin{array}{l}0=\frac{1}{4\pi {\epsilon }_0}\cdot \frac{q_1}{s}+\frac{1}{4\pi {\epsilon }_0}\cdot \frac{q_2}{(s-d)}\Rightarrow \frac{1}{4\pi {\epsilon }_0}\cdot \frac{q_1}{s}=-\frac{1}{4\pi {\epsilon }_0}\cdot \frac{q_2}{(s-d)}\\ \Rightarrow \frac{q_1}{s}=-\frac{q_2}{(s-d)}\\ \Rightarrow \frac{5\times {10}^{-8}}{s}=-\frac{(-3\times {10}^{-8})}{(s-0.16)}\\ \Rightarrow 5(s-0.16)=3s\\ \Rightarrow 0.8=2s\Rightarrow s=0.4m=40cm\end{array}$

Therefore, at a distance of 40 cm from the positive charge outside the system of charges, the potential is zero.