Please be patient as the PDF generation may take upto a minute.

A particle is moving along the x-axis under a conservative force and its potential energy U varies with x co-ordinate as shown in the figure. Then force is positive at:
                           
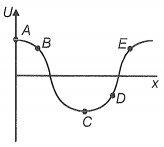
(1)  A
(2)  C, D 
(3)  B
(4)  D, E

The potential energy \(\mathrm{U}\) of a system is given by $\mathrm{U}=$ $\mathrm{A}$ $-$ ${\mathrm{Bx}}^2$ (where \(\mathrm{x}\) is the position of its particle and \(\mathrm{A},\) \(\mathrm{B}\) are constants). The magnitude of the force acting on the particle is:
1. constant
2. proportional to \(\mathrm{x}\)
3. proportional to ${\mathrm{x}}^2$
4. proportional to $\left(\frac{1}{\mathrm{x}}\right)$

If U is the potential energy of a particle and x is its displacement, then in the position of stable equilibrium,
1.  $\frac{\mathrm{dU}}{\mathrm{dx}} = 0 \mathrm{and} \frac{{\mathrm{d}}^2\mathrm{U}}{{\mathrm{dx}}^2} > 0$
2.  $\frac{\mathrm{dU}}{\mathrm{dx}} = 0 \mathrm{and} \frac{{\mathrm{d}}^2\mathrm{U}}{{\mathrm{dx}}^2} < 0$
3.  $\frac{\mathrm{dU}}{\mathrm{dx}} = 0 \mathrm{and} \frac{{\mathrm{d}}^2\mathrm{U}}{{\mathrm{dx}}^2} = 0$
4.  All of these

The given plot shows the variation of U, the potential energy of interaction between two particles with the distance separating them, r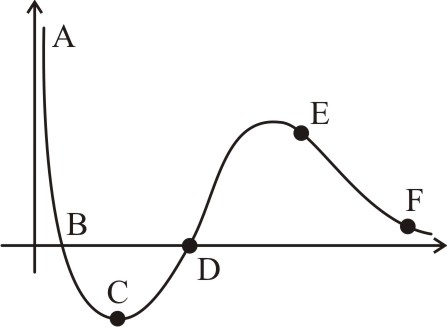

1. B and D are equilibrium points
2. C is a point of stable equlibrium
3. The force of interaction between the two particles is attractive between points C and D and repulsive between points D and E on the curve.
4. The force of interaction between the particles is attractive between points E and F on the curve.

A particle located in a one-dimensional potential field has its potential energy function as $U\left(x\right)=\frac{a}{x^4}-\frac{b}{x^2}$, where a and b are positive constants. The position of equilibrium x corresponds to 
1. $\frac{b}{2a}$
2. $\sqrt{\frac{2a}{b}}$
3. $\sqrt{\frac{2b}{a}}$
4. $\frac{a}{2b}$

In the figure potential energy of a particle varies with \(\mathrm{x}\)-co-ordinates. The particle moves under the effect of conservative force along the \(\mathrm{x}\)-axis.
    

The points of maximum and minimum attraction in the curve between potential energy (U) and distance (r) of a diatomic molecules are respectively -

(1) S and R
(2) T and S
(3) R and S
(4) S and T

A particle free to move along the x-axis has potential energy given by $U\left(x\right)=k[1-e^{-x^2}]$ for $-\infty \le x\le +\infty$, where k is a positive constant of appropriate dimensions. Then 
(1) At point away from the origin, the particle is in unstable equilibrium
(2) For any finite non-zero value of x, there is a force directed away from the origin
(3) If its total mechanical energy is k/2, it has its minimum kinetic energy at the origin
(4) For small displacements from x = 0, the motion is simple harmonic