Please be patient as the PDF generation may take upto a minute.

If the angle between the vector $\overrightarrow{A} and \overrightarrow{B}$ is  $\theta$, the value of the product $\left(\overrightarrow{B}\times \overrightarrow{A}\right).\overrightarrow{A}$ is equal to:
1. $B{A}^2 \cos \theta$
2. $B{A}^2 \sin \theta$
3. $B{A}^2 \sin \theta \cos \theta$
4. zero

Two forces are such that the sum of their magnitudes is \(18~\text{N}\) and their resultant is perpendicular to the smaller force and the magnitude of the resultant is \(12~\text{N}\). Then the magnitudes of the forces will be:
1. \(12~\text{N}, 6~\text{N}\)
2. \(13~\text{N}, 5~\text{N}\)
3. \(10~\text{N}, 8~\text{N}\)
4. \(16~\text{N}, 2~\text{N}\)

If the magnitude of the sum of two vectors is equal to the magnitude of the difference between the two vectors, the angle between these vectors is:
1. \(90^{\circ}\)
2. \(45^{\circ}\)
3. \(180^{\circ}\)
4. \(0^{\circ}\)

The resultant of two vectors A and B is perpendicular to the vector A and its magnitude is equal to half the magnitude of vector B. The angle between A and B is -
                               
1. $120°$
2.  $150°$
3.  $135°$
4.  None of these

What is the resultant of three coplanar forces: $300 \mathrm{N}$ at $0°$, $400 \mathrm{N}$ at $30°$ and $400 \mathrm{N}$ at $150°$?
1.  $500 \mathrm{N}$
2.  $700 \mathrm{N}$
3.  $1100 \mathrm{N}$
4.  $300 \mathrm{N}$

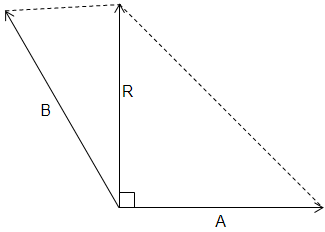
For the figure -
 
1.  A+B=C
2.  B+C=A
3.  C+A=B
4.  A+B+C=0

The two vectors $\mathrm{A}=2\hat{\mathrm{i}}+\hat{\mathrm{j}}+3\hat{\mathrm{k}}$ and $\mathrm{B}=7\hat{\mathrm{i}}-5\hat{\mathrm{j}}-3\hat{\mathrm{k}}$ are -
1.  parallel
2.  perpendicular
3.  anti-parallel
4.  None of these

A vector A points, vertically upward and, B points towards north. The vector product $\mathrm{A}\times \mathrm{B}$ is -
1.  along west
2.  along east
3.  zero
4.  vertically downward

The vector having a magnitude of 10 and perpendicular to the vector $3\hat{\mathrm{i}}-4\hat{\mathrm{j}}$ is- 
1. $4\hat{\mathrm{i}}-3\hat{\mathrm{j}}$
2. $5\sqrt{2} \hat{\mathrm{i}}-5\sqrt{2} \hat{\mathrm{j}}$
3. $8\hat{\mathrm{i}}+6\hat{\mathrm{j}}$
4.  $8\hat{\mathrm{i}}-6\hat{\mathrm{j}}$

If \(\overrightarrow{A}={2}\hat{i}+\hat{j}\;{\&}\;\overrightarrow{B}=\hat{i}{-}\hat{j},\)  then the components of \(\overrightarrow {A}\) along with \(\overrightarrow {B}\) & perpendicular to \(\overrightarrow {B}\) respectively will be:
1. \(\frac{\hat{i} - \hat{j}}{2} ,~\frac{3}{2}\left(\hat i+\hat j\right)\)
2. \(\frac{\hat{i} - \hat{j}}{2} ,~-\frac{2}{3}\left(\hat i+\hat j\right)\)
3. \(\frac{\hat{i} - \hat{j}}{2} ,~-\frac{3}{2}\left(\hat i-\hat j\right)\)
4. \(\frac{\hat{i} - \hat{j}}{2} ,~\frac{2}{3}\left(\hat i-\hat j\right)\)

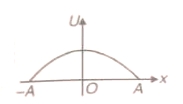
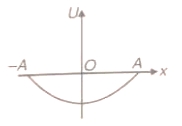
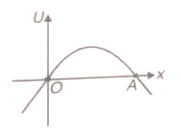
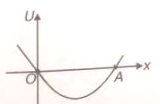
Which of the following represented to U-x graph of U = $\frac{1}{2}K(A^2 - x^2) ? (\mathrm{K}>0)$
1. 
2. 
3. 
4. 

If curve between acceleration ($\mathrm{\alpha }$) and position (x) is a straight line as shown. Then acceleration is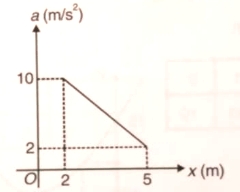

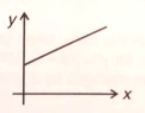
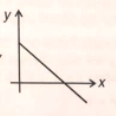
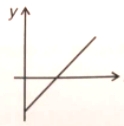
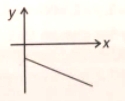
$1. \frac{40}{3}-\frac{8}{3}\mathrm{x}$
$2. \frac{46}{3}-\frac{8}{3}\mathrm{x}$
$3. \frac{5}{6}-\frac{8}{3}\mathrm{x}$
$4. \frac{1}{3}-\frac{5}{2}\mathrm{x}$

The straight line y = -2x + 5 is best represented by
1. 
2. 
3. 
4. 

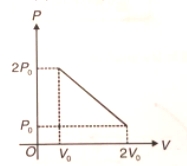
1. \(2P_0 - \frac{P_0V}{V_0}\)
2. \(3P_0 - \frac{P_0V}{V_0}\)
3. \(P_0 - \frac{P_0V}{V_0}\)
4. \(4P_0 - \frac{P_0V}{V_0}\)

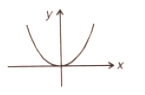
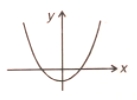
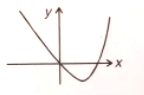
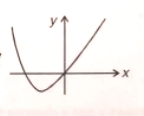
The parabola y = x${}^2$ + 2x is represented by
1. 
2. 
3. 
4.

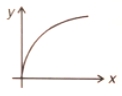
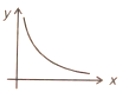
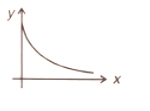
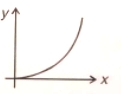
The relationship y = $\frac{3}{\mathrm{x}}$ is best represented as 
1. 
2. 
3. 
4. 

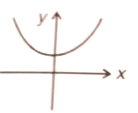
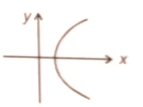
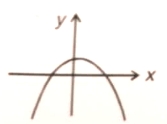
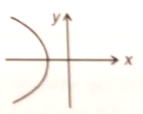
The parabola y = x${}^2$ + 1 is represented by
1. 
2. 
3. 
4. 
 

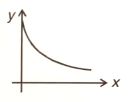
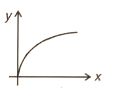
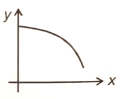
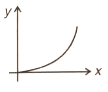
The slope of a curve between y and x changes from 0 to -5 as x changes from 0 to 5. Which of the following curve best represents the above mentioned curve?
1. 
2. 
3. 
4. 

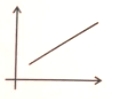
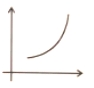
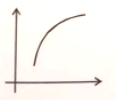
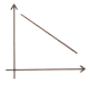
Which of the following graph has a positive, decreasing slope?
1. 
2. 
3. 
4. 

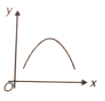
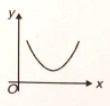
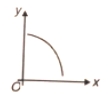
If slope of curve is first positive, then zero and after that became negative, so best represented of following graph which satisfied above condition
1. 
2. 
3. 
4.